We need to explore the interesting essence of quadratic equations in this post. We will normally concentrate on making the solution to. Quadratic equations are a significant component of algebra and are fundamental in many branches of science and mathematics. To improve our comprehending power, we will go over the process for solving this equation, talking about its uses, and present instances throughout this piece.
A quadratic equation: what is it?
Second-degree polynomial equating, or those having variables raised to the power of 2, are called as quadratic equations. A quadratic equation could be written in the normal form as ax^2 + bx + c = 0, where x is a variable we can solving for and a, b, and c were the coefficients.
Recognizing the General Form
The coefficients in the particular equating, 4x ^ 2 – 5x – 12 = 0, are a equals 4, b equals -5, and c equals -12. We can see that the equation follows the normal form of a quadratic equation by rearrange to it.
Qualities of Quadratic Formulas
Certain features of quadratic equations are essential to understanding and solving them. These consist of having symmetrical characteristics, a vertex that indicates the minimum or maximum point, and a parabolic form. Gaining knowledge of these attributes enables us to comprehend their behavior and useful applications.
Factoring Technique
Using the factoring method, the quadratic equation is divided into its constituent parts, each of which can be solved separately. This approach, meanwhile, is only effective in factorable equations.
To use the factors method to solve 4x2 – 5x – 12 = 0, we must first know two binomials which multiply together to give the quadratic equation. The factoring form in this example would be (2x + 3)(2x – 4) = 0.
2 equations coming from setting any factor to zero: 2x + 3 = 0 or 2x – 4 = 0. We can find the values of x by solving these equations. Therefore, the equation’s solutions are x = -3/2 and x = 2.
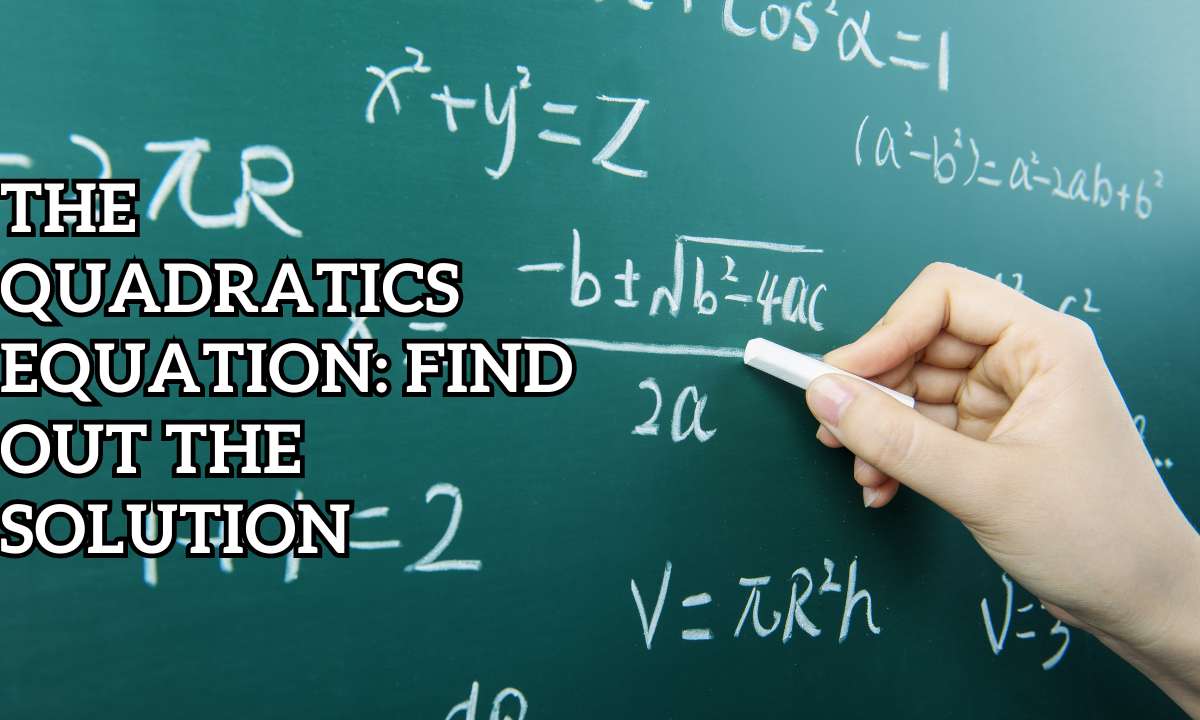
Formula for Quadratic Formula
An alternative approach to solving quadratic problems is offered by the quadratic formula. Even in cases where factoring an equation is difficult, this effective technique ensures that the answers will be found.
The quadratic method could be given to the issue of given equates to 0.
x equals (-b± √(b^2 – 4ac)) division by 2a.
We can find the solutions for x by entering the variables a = 4, b = -5, and c = -12 into the formula. Upon completing the required computations, we determine that x = -3/2 and x = 2.
In summary
Finally, we have learned different methods for solving the quad equation. We could determine the values of x that fill in the equation by taking the quadratic formula or the factoring process. In addition, we now know that quadratic equations have practical uses in disciplines like physics, engineering, and projectile motion. Comprehending quadratic equations is crucial for resolving an extensive array of mathematical issues and offers significant understanding into the actions of diverse occurrences.
Also, Read About:-